Basic HTML Version
222
reduced by a factor of 2 then the intrinsic viscosity is reduced by a factor of
2
1.8
= 3.48. Thus, small changes in M are easily detected by a comparatively
larger change in the intrinsic viscosity (for rods).
6.1.5.
Intrinsic viscosity of
randomly
coiled polymers
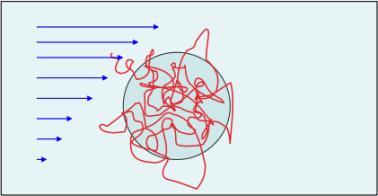
For random coils the situation resembles, perhaps non-intuitively, that of solid
spheres. This is because dissolved randomly coiled molecules in fact are very
open structures holding a large volume of bound water. This water ‘flows’ and
rotates with the molecule as the molecule is transported in a shear regime.
Moreover, the extent of interpenetration is negligible. Thus, randomly coiled
molecules behave as water-filled (‘non-draining’), solid spheres.
The major point is that the equivalent hydrodynamic radius of the sphere (R
e
)
can be taken to be proportional to the radius of gyration:
R
e
=
ξ
R
G
We can thus apply the formalism of solid spheres (Einstein’s equation), which
requires calculation of the specific hydrodynamic volume (v
h
). This is
straightforward:
Volume per sphere:
v'= 4
3
π
R
e
3
Volume per gram (hydrodynamic volume):
v
h
=
v
'
N
Avo
M
=
4
3
πξ
3
R
G
3
N
Avo
M
This expression can be inserted directly into Einstein’s equation:

